The Layman’s Summary
Working Name: Black Donut
Descriptive Name: Toroidal Black Holes
Date Conceived: 19-August-2013
Concept
I had been tossing this around for about a week when I finally came to this conclusion, which is when I marked the date. I was exploring the possible application of super/hyper-dense materials in propulsion. That is, I was wondering if you could use the gravitational pull of very dense materials to slingshot a human-made object in space. If a small, high-density phenomenon were artificially generated, then it would rapidly expand and evaporate, both characteristics being useful. I explored (what I think is) a practical way of doing this, but there are a lot of questions yet to be answered about it.
Anyway, in following one branch of this concept, I arrived at black holes almost inevitably. Sparing you the details, the process, and the convoluted math (it’s actually pretty straight-forward), I came to the following set of realizations.
1. Radius Never Equals Zero: The equation describing the relationship between volume and gravity goes to a gravitational value of a given mass over a radius of zero, which translates to infinity, a meaningless value in science. Consider the speed of light (c). Mass never quite gets there. It can’t without becoming energy. In the same vein, the radius of a black hole can never equal zero without the material within it turning into something else. Since infinity is an essentially meaningless concept, I use a calculus principle called a limit. Thus, rather than volume being equal to zero, volume approaches but never reaches zero (after all, radius is cubed, which is a steep curve in favor of staying relevant). My assumption is that radius never actually equals zero, but instead approaches an asymptote that it never quite reaches.
2. Black holes are also pulled outward: Objects in space spin. There are lots of forces trying to crush a star and trying to tear it apart. Gravity squeezes stars down while their internal nuclear reactions try to blow them apart. Centripetal force plays a small role in trying to pull the star apart. Black holes spin. As they collapse from large stars, their nuclear fuel consumed, their radius reduces. Like an ice skater pulling in his arms, the object spins faster. A radius change from arms outstretched to shoulder width results in a noticeable change in angular velocity. A radius change of an object the size of a very large star to the size of… –well, we’re not really sure, since we can really only measure the event horizon… but very small, let’s say an orange to have something to compare it to– is an enormous change in angular velocity. As other orbiting material falls into it, they impart additional momentum and the black hole spins yet faster and faster. There is presumably an upper limit to how fast it can spin (the angular velocity); the speed of light (c), of course.
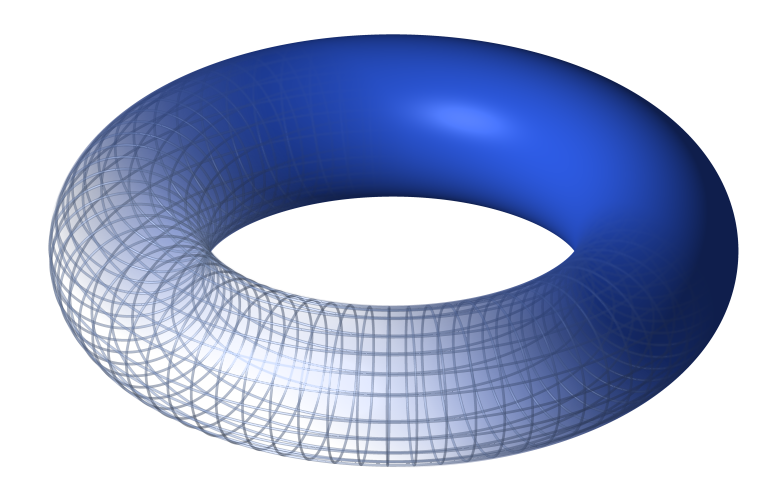
3. Black Donut: There is a ratio of the relationship between the effect of normal gravity and the opposing effect of centripetal force. The approach to the asymptotes is steep, so there are regions wherein the effect of one force might be slightly higher than the other. I speculated that the material of a spherical black hole might be pulled into a torus around the radius.
This may not happen gradually but perhaps in rapid succession, layers snapping away into their new orbits. It is also possible that it happens very slowly, transitional stages becoming the stable shapes.
4. Null space: As normal space attempts to pass through the center of the torus, it is pulled equally in every direction on the plane away from the center in a region shaped like an oblong fishing floater. It is difficult to understand what this might look like inside, but my best guess is two part:
1. A region outside of space-time, as space-time has been pulled/torn open. That is, this space is not in our universe. This space might look like what null space looked like before the Big Bang. I have some ideas (really just one) about what null space might actually be, but that’s for another post.
2. An equi-potential region where forces are neutralized by complementary opposite forces and space-time is distorted almost to the point of null space, but not quite, where time is nearly frozen.
5. Vibration: As the donut spins, the inside should attempt to spin faster than the outside (particularly when not feeding). This is because material closer to the center of the phenomenon has a smaller radius. As discussed, a smaller radius leads to a higher angular velocity. The material on the inside, however, is still in contact with the outer portion of the ring due to the nearly infinite density of the phenomenon. I think that this makes it possible for a friction differential/potential to build up like a wrinkle in a rubber band or a guitar string pulled but not released. Attempting to normalize, this potential is released and a wave travels around the ring (symmetrically) and back as it over- and then under-corrects for this temporary deformity.
Imagine an ellipse in the vertical that responds to that deformation by then becoming an ellipse in the horizontal, ad infinitum. This presumably regular vibration (as occurs in closed vibrating systems like strings, drums, and triangles) should be detectable in its gravitational imprint on surrounding material. This would have a few effects:
1. Spinning at nearly c with a comparably high frequency (how often peaks and valleys occur), this is almost smooth to observation.
2. There would be different patterns/frequencies for different donuts.
3. Such a vibration would distort the shape, nature, and behavior of the null/almost-null space at the center. The effect of this is difficult to picture.
4. Such a vibration would also distort the shape, nature, and behavior of the normal material surrounding the donut. This effect should be easily observable.
Developed
I thought that there was a chance that this was correct but I didn’t want to be confused with these people, so I was reluctant to share it until I could verify the claims I was making. That might have had to wait until late next year.
As with most things that catch my interest, I know more about black holes than most people, but not enough to be considered a serious astrophysics hobbyist (yes, they exist). I was researching this, trying to see if I was at least barking up the right tree when I learned about New Zealander Roy Kerr and his Kerr Black Hole. He came to the same conclusion in 1960; that at least some black holes must have a torus shape. I learned this on August 21st, just two days after I figured it out. Gotta admit, pretty neat. So I came up with this one second, not first. I’m behind schedule by 50 years.
Given his knowledge and that of his colleagues, he was able to make several more predictions to include the presence of neutrons and a charge/polarity (the latter conclusion I think I would also have reached). In fact, the wormhole theory is tied to the null space in the torus. I don’t know if this means that I’m smart enough to figure this kind of thing out, or not smart enough to have known it already.
Implications
The ring/donut vibrates in a regular manner. In addition, like any other body with mass, it can be orbited by less massive bodies (typically the disparity is enormous). In the case of the torus, some of those orbits pass through the center when traveling along their orbits. Recall that this center in the black donut is null space. That is, an orbiting body enters and (presumably) exits null space routinely. This behavior is reminiscent of a quantum string and the behavior of other quantum particles, which I wasn’t sure I understood or believed until I followed this thought experiment.
If it can be shown mathematically to be correct (and, despite having taken a great deal of math, it remains the lesser of my abilities), then there may be some connection finally between the very very very small and the very very very big. Again, none of this was my goal. I just kind of got here by accident.
Application
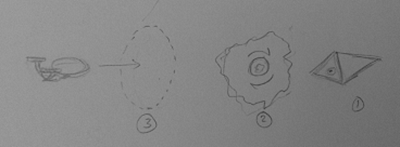
The original purpose of this line of thought was to see if a temporary region of dense matter (not a true black hole) could be used to slingshot a vessel at a high velocity. I envisioned a uranium donut packed tightly in carbon in a container made of who-knows-what. Launched from the front of a vessel while spinning and detonated in three places, a temporary super dense donut might be created for just a tenth of a second.
Here’s a rough sketch of what it might look like:
And how it might happen:
If a vessel or object could survive the gravitational differential (different parts of the vessel being different distances to the center of the phenomenon) and the acceleration from zero to very very fast without turning into cheese wiz, then by the time it got to the center, the phenomenon would have evaporated and the craft would maintain its last velocity. Don’t ask me how to stop.
So, what do you think? Is there any connection between a black hole/donut and a quantum string? Would being slung through a rapidly evaporating ring of dense material turn anything going through it into a spray from a shower head?
Keep an eye out in December for a video explaining all of this. As the central project in an honors course, I’m making a few CAD animations that explain and explore all of this. A five minute video should be less grueling than reading all of this.
Cya next time,
-CG
References
You can look this material up on Wikipedia, but I largely based my thinking on the following:
Walker, J. (2008) Fundamentals of Physics. 8th Edition. Halliday and Resnick. Chapter 13: Gravitation and Chapter 16-13: Standing Waves and Resonance
Wolfram Alpha (links in post)